

|
In this section, we give an algebraic treatment of these topics.
The proofs may be obtained by clicking on the link below the statement of each theorem.
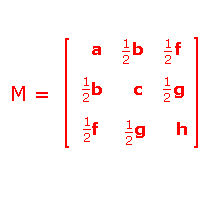
A plane conic has an equation of the form
ax2+bxy +cy2+fx+gy+h=0.
In terms of homogeneous coordinates, this becomes
ax2+bxy +cy2+fxz+gyz+hz2=0
which can be written as
xTMx=0
where x=(x,y,z), and M is a symmetric 3x3 matrix.
For a non-degenerate conic, M must be non-singular and have eigenvalues of different sign.
Note that, if a conic contains three (distinct) collinear points, then it must be degenerate.
Definition
If C: xTMx=0 is a non-degenerate conic and
U=[u] is any point,
then the algebraic polar of U with respect to C is the line
uTMx=0.
Note that, as M is non-singular, we cannot have uTM=0, so that the line always exists.
A line L has an equation aTx=0.
Now, uTMx=0 and aTx=0
give the same line if and only if [u]=[M-1a].
Thus L is the polar of a unique point U=[u].
Definition
If C: xTMx=0 is a non-degenerate conic and
L is any line,
then the algebraic pole of L with respect to C
is the point U=[u] such that L has equation
uTMx=0.
Remark
If L has equation aTx=0, then, as we have seen,
the pole of L is U=[M-1a].
Theorem 1
If C: xTMx=0 is a non-degenerate conic and
U is any point on C,
then the algebraic polar of U with respect to C is the tangent to C at U
.
We now show that the algebraic polar coincides with the geometrical polar.
Theorem 2
Suppose that C: xTMx=0 is a non-degenerate conic and
that VW is a chord of C passing through a fixed point U.
Then the tangents at V and W meet on the algebraic polar of U with respect to C.
It follows readily that the algebraic and geometric poles of a line coincide.
We now have the idea of duality defined in algebraic terms.
Theorem 3 La Hire's Theorem
From certain points in the plane, it is possible to draw tangents to the conic C.
Theorem 4
Together, Theorems 1, 2 and 4 establish
Of course, the second is really a special case of the first
(but we proved it as Theorem 1 to help with the proof of Theorem 2).
We now show that the dual of a conic with respect to a fixed conic is actaully another conic.
Theorem 5
The fundamental result is that duality preserves incidence.
Suppose that C: xTMx=0 is a non-degenerate conic and that
U and V are any points.
Then U lies on the polar of V
if and only if V lies on the polar of U.
Provided the point does not lie on the conic, there will be exactly two tangents, as the following theorem shows.
Suppose that C: xTMx=0 is a non-degenerate conic and that
U is any point.
Then the pair of tangents to C from U has equation
uTMu xTMx
=uTMx uTMx .
 Joachimsthal's Formulae
Joachimsthal's Formulae
Suppose that C: xTMx=0 is a non-degenerate conic. Then
Tell me about Joachimsthal
There is a macro to draw the dual conic in the conics toolbar.
Suppose that C: xTMx=0 and
D: xTNx=0 are non-degenerate conics.
Then the dual of D with respect to C is the non-degenerate conic with equation
xTMN-1Mx=0.
| Main Conics Page | Geometry Page | Main Cabri Page |