


|  |
The Inversion TheoremLet C be a circle with centre O.For any object X, let X' denote the inverse of X with respect to C.
|
|
Proof1. Suppose that P is a point on L (other that Å or O).Then, by clause (1) of the definition of inversion, P' lies on L. Since inversion in C interchanges O and Å, the result follows.
|
|
|
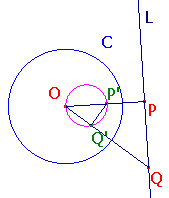
2.
Let P be the foot of the perpendicular from O to L. Suppose that Q is a point on L (other that Å or P), so <QPO is right. By the Equal Angles Theorem, <QPO = <OQ'P', so <OQ'P' is a right angle. Then Q' lies on the semicircle on OP' as diameter. since Å maps to O, we are done. 3. As inversion has order 2, (3) is equivalent to (2).
|
 |
|
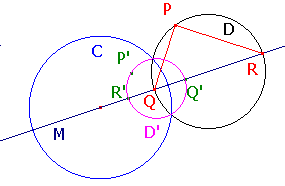
4. Let M denote the line joining O to the centre of D. Suppose this cuts D at Q and R, so that QR is a diameter of D. Now let P be a point on D, other than Q or R. As an angle in a semicircle <QPR is a rigth angle. As Q and R are on D, Q' and R' will lie on D'. By the Corollary to the Equal Angles Theorem, <:QPR = <R'P'Q', so the latter is also a right angle. Thus, P' lies on the circle on Q'R' as diameter. It follows that D' is precisely this circle. |
 |
CabriJava illustrations of cases 2 and 4
| Main Inversive Page | Main CabriJava Page | MainCabri Page |